Ok, I’m jumping on my soap box . . . well sort of.
My students are currently working on Mean, Median, and Mode in class. It is a tested standard.
For those who need a review:
- Mean: Average – You add all the numbers in the set of data and the divide by how many numbers there are.
- Median: Middle Number – List the numbers in order and find the middle number.
- Mode: Most Often – What is the number (letter, word, etc.) that appears most often.
My students have been working on mode and median and they have a good understanding on how to find those in a set of data. That can be done without a calculator. It’s just a matter of rewriting the numbers and looking at the data.
it’s the mean that is so MEAN.
Actually, it’s not the mean, it’s the state that doesn’t allow use of a calculator that’s mean.
(Do you think I’m mean to confuse you about which mean I mean whenever I mention mean?)
There are tested standards for addition of numbers. I understand not allowing a calculator for this because we need to make sure that students understand the process of adding.
There are tested standards for division of numbers. I understand not allowing a calculator for this because we need to make sure that students understand the process of dividing.
However, not allowing a calculator to find the mean or average of data is down right MEAN! (and kinda stupid)
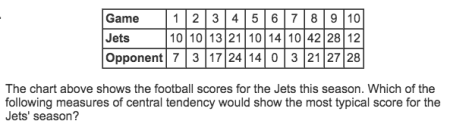
If a student messes up on finding the mean of the following data . . . .
So to find the mean of the Jets score you have to add 10 and 10 and 13 and 21 and 10 and 14 and 10 and 42 and 28 and 12, then after you add then you have to divide by 10. You also have to find the mean of the opponent’s score by adding 7 and 2 and 17 and 24 and 14 and 0 and 3 and 21 and 27 and 28, then dividing by 10 (anyway I think that’s the number because one of them is 0). Anyway, now that I’ve done all of that I hope that I solved it correctly . . . .
Do you understand my point? Are we trying to see if they can add all of the numbers or are we trying to see if they understand how to find the mean?
Why can’t they use a calculator to find mean. Let’s look. Is it possible that they may accidentally press the wrong number in the calculator? YES!! THEY DO IT ALL THE TIME!!!
I guess what’s frustrating is that we are so focused at the state level of not allowing calculators that many students just give up the moment they see all the numbers in the problem listed above and just take a guess. What is that guess showing? That I’m a bad teacher? That the students aren’t learning? No, it’s showing that the students think that the question could possibly be testing the wrong standard.
If you want to see if students understand how to find the mean, allow them to use a calculator to find the answer quicker.
HEY! it’s the REAL WORLD!!!
Ask someone in the legislation to find the mean of some numbers (like the average dollar amount that they raise for campaigning), I bet they grab a calculator. IT’S THE REAL WORLD!!!
Ask scientists what the mean magnitude of earthquakes in Oklahoma (yes, we have them) have been over the past month. Guess what, they will GRAB A CALCULATOR. That’s the real world.
So why do we deprive students of a TOOL when finding more than just if they can add or divide? Why can’t they use the calculator?
What do you think about this? Please share your comments with me. Also, SHARE THIS POST WITH YOUR LEGISLATOR so they can begin to understand what should and should not be tested.